Langkau ke kandungan utama

Pressure And Depth (2)

Assalamualaikum w.b.t, saya MOHAMMAD ARIFF FARHAN BIN ZULKARIA (13DEM19F1035) dari kelas DEM2S1 ingin menceritakan tentang PRESSURE AND DEPTH yang dibentangkan oleh ENCIK ROSHAIZUL NIZAM BIN SANI.

If your ears have ever popped on a plane flight or ached during a deep dive in a swimming pool, you have experienced the effect of depth on pressure in a fluid. At the Earth’s surface, the air pressure exerted on you is a result of the weight of air above you. This pressure is reduced as you climb up in altitude and the weight of air above you decreases. Under water, the pressure exerted on you increases with increasing depth. In this case, the pressure being exerted upon you is a result of both the weight of water above you and that of the atmosphere above you. You may notice an air pressure change on an elevator ride that transports you many stories, but you need only dive a meter or so below the surface of a pool to feel a pressure increase. The difference is that water is much denser than air, about 775 times as dense.
Consider the container. Its bottom supports the weight of the fluid in it. Let us calculate the pressure exerted on the bottom by the weight of the fluid. That pressure is the weight of the fluid \text{mg} divided by the area A supporting it (the area of the bottom of the container):
P=\frac{\text{mg}}{A}.
We can find the mass of the fluid from its volume and density:
m=\mathrm{\rho V}.
The volume of the fluid V is related to the dimensions of the container. It is
V=\text{Ah},
where A is the cross-sectional area and h is the depth. Combining the last two equations gives
m=\rho \text{Ah}.
If we enter this into the expression for pressure, we obtain
P=\frac{\left(\rho \text{Ah}\right)g}{A}.
The area cancels, and rearranging the variables yields
P=\mathrm{h\rho g}.
This value is the pressure due to the weight of a fluid. The equation has general validity beyond the special conditions under which it is derived here. Even if the container were not there, the surrounding fluid would still exert this pressure, keeping the fluid static. Thus the equation P=\mathrm{h\rho g} represents the pressure due to the weight of any fluid of average density \rho at any depth h below its surface. For liquids, which are nearly incompressible, this equation holds to great depths. For gases, which are quite compressible, one can apply this equation as long as the density changes are small over the depth considered.Illustrates this situation.
The bottom of this container supports the entire weight of the fluid in it. The vertical sides cannot exert an upward force on the fluid (since it cannot withstand a shearing force), and so the bottom must support it all.

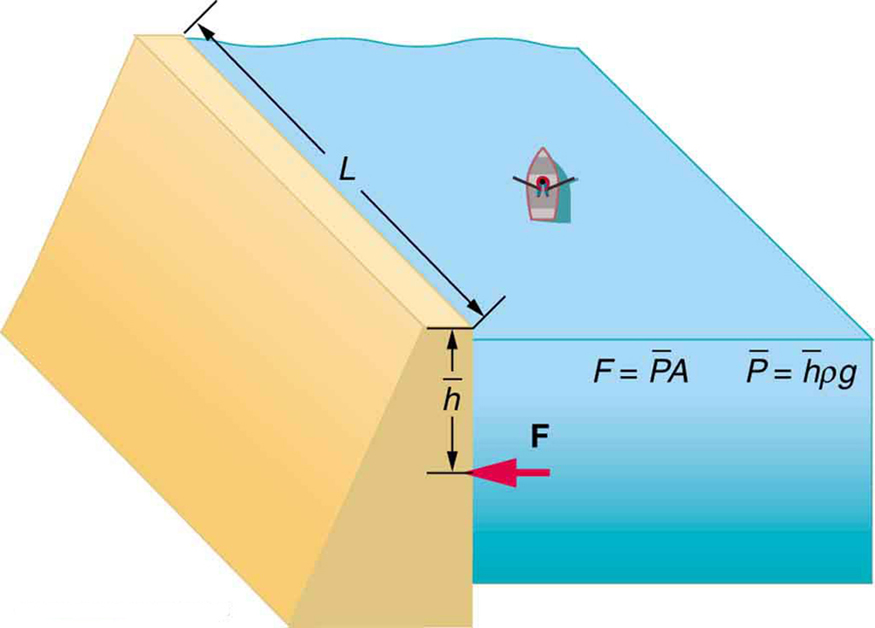
Calculating the Average Pressure and Force Exerted: What Force Must a Dam Withstand?
We calculated the mass of water in a large reservoir. We will now consider the pressure and force acting on the dam retaining water. The dam is 500 m wide, and the water is 80.0 m deep at the dam. (a) What is the average pressure on the dam due to the water? (b) Calculate the force exerted against the dam and compare it with the weight of water in the dam (previously found to be 1.96×{10}^{13}\phantom{\rule{0.25em}{0ex}}\text{N}).
Strategy for (a)
The average pressure \overline{P}
due to the weight of the water is the pressure at the average depth \overline{h}
of 40.0 m, since pressure increases linearly with depth.
Solution for (a)
The average pressure due to the weight of a fluid is
\overline{P}=\overline{h}\mathrm{\rho g}.
Entering the density of water from and taking \overline{h} to be the average depth of 40.0 m, we obtain
\begin{array}{lll}\overline{P}& =& \left(\text{40.0 m}\right)\left({\text{10}}^{3}\phantom{\rule{0.25em}{0ex}}\frac{\text{kg}}{{\text{m}}^{3}}\right)\left(9.80\phantom{\rule{0.25em}{0ex}}\frac{\text{m}}{{\text{s}}^{2}}\right)\\ & =& 3.92×{\text{10}}^{5}\phantom{\rule{0.25em}{0ex}}\frac{\text{N}}{{\text{m}}^{2}}=\text{392 kPa.}\end{array}
Strategy for (b)
The force exerted on the dam by the water is the average pressure times the area of contact:
F=\overline{P}A.
Solution for (b)
We have already found the value for \overline{P}. The area of the dam is A=\text{80.0 m}×\text{500 m}=4.00×{\text{10}}^{4}\phantom{\rule{0.25em}{0ex}}{\text{m}}^{2}, so that
\begin{array}{lll}F& =& \left(3.92×{\text{10}}^{5}\phantom{\rule{0.25em}{0ex}}{\text{N/m}}^{2}\right)\left(4.00×{\text{10}}^{4}\phantom{\rule{0.25em}{0ex}}{\text{m}}^{2}\right)\\ & =& 1.57×{\text{10}}^{\text{10}}\phantom{\rule{0.25em}{0ex}}\text{N.}\end{array}
Discussion
Although this force seems large, it is small compared with the 1.96×{\text{10}}^{\text{13}}\phantom{\rule{0.25em}{0ex}}\text{N} weight of the water in the reservoir—in fact, it is only
0.0800% of the weight. Note that the pressure found in part (a) is completely independent of the width and length of the lake—it depends only on its average depth at the dam. Thus the force depends only on the water’s average depth and the dimensions of the dam, not on the horizontal extent of the reservoir. In the diagram, the thickness of the dam increases with depth to balance the increasing force due to the increasing pressure.epth to balance the increasing force due to the increasing pressure.
The dam must withstand the force exerted against it by the water it retains. This force is small compared with the weight of the water behind the dam.
A two-dimensional view of a dam with dimensions L and h is shown. Force F at h is shown by a horizontal arrow. The force F exerted by water on the dam is F equals average pressure p bar into area A and pressure in turn is average height h bar into density rho into acceleration due to gravity g.
We also can see about pressure and depth in link https://www.youtube.com/watch?v=5EWjlpc0S00 and https://www.youtube.com/watch?v=K5g6P8-GmBg

That's all from me to present about pressure and depth.

Ulasan

Catat Ulasan

Catatan popular daripada blog ini

EKSPERIMEN 1: SIFAT FIZIKAL CECAIR

Assalammualaikum w.b.t.      Saya MUHAMMAD AMIRUL AKMAL sebagai wakil pelajar dari kelas DEM2S1  di POLITEKNIK SULTAN MIZAN ZAINAL ABIDIN (PSMZA) akan menceritakan tentang Termofluid. T ERMOFLUID  merupakan subjek yang kerap digunapakai di dalam teknologi kejuruteraan dan sains di bagi menyelesaikan beberapa permasalahan. Bendalir mekanik juga bersifat rumit dan kadang-kala ia hanya boleh diselesaikan dengan kiraan dan formula tertentu berdasarkan prinsip bendalir mekanik, terutamanya dengan menggunakan kaedah dinamik bendalir berkomputeran (computational fluid dynamics). Kaedah ini dapat menyelesaikan masalah bendalir dengan kaedah menerusi pemvisualan dan menganalisa aliran bendalir. Pada 10.12.19 yang lalu,telah diberikan satu tugasan iaitu eksperimen tentang sifat fizikal bendalir. Alatan yang digunakan: 1) Penimbang Skala Digital 2) Bikar 3) Minyak Masak 4) Minyak Enjin Prosedur: 1) Tekan suis ON pada penimbang skala digital 2) Letakkan bikar di atas penimbang
Catat Ulasan
Baca lagi

BAB 1 : CONCEPTUAL PRINCIPAL OF THERMOFLUIDS

BAB 1 : CONCEPTUAL PRINCIPAL OF                THERMOFLUIDS Assalammualaikum w.b.t.      Saya Mohamad Shahril Atiq Bin Salleh😎 wakil pelajar dari kelas DEM2S1 POLITEKNIK SULTAN MIZAN ZAINAL ABIDIN (PSMZA). Kini saya adalah pelajar semester 2 sesi Jun 2019 dalam bidang Diploma Kejuruteraan Mekatronik (DEM). Bidang ini selalu kita dengar sebagai satu bidang yang agak susah😐, apatah lagi kebanyakkan subjeknya berkaitan dengan pengiraan dan fakta. Oleh demikian, saya diberi tugas untuk membuat rumusan  yang berkaitan dengan tajuk pertama dalam subjek Thermofluids bagi memudahkan pelajar memahaminya👍.       Okay gais, secara keseluruhannya bab 1 ini boleh dikatakan hampir 70% berkaitan pengiraan. So kalau korang yang mantopp dalam pengiraan tu, mustahil laa tak boleh nak score an an😏. Kalau ada yang lemah dalam pengiraan tu, korang masih boleh berusaha lagi, yang penting jangan patah semangat. Takde apa yang senang gais, nak berjaya kenalah usaha🤗.       Kembali kepa
Catat Ulasan
Baca lagi

BAB 2: FLUID APPLICATION PART 1

       BAB 2 : FLUID APPLICATION PART 1 Assalamualaikum w.m.t              Saya  FARAH HAZIQAH AUNI BINTI FAIZAL 😘 wakil pelajar dari kelas DEM2A1 di  POLITEKNIK SULTAN MIZAN ZAINAL ABIDIN  yang terletak di Terengganu. Saya ingin menceritakan tentang hukum paskal dan jek hydraulik. PRINSIP DAN HIDRAULIK PASKAL 💙 Prinsip paskal menyatakan bahawa tekanan yang dikenakan keatas sesuatu cecair boleh dipindahkan  ke seluruh cecair itu dengan seragam. prinsip paskal juga dikenali sebagai prinsip pemindahan cecair dalam cecair. prinsip pemindahan tekanan dalam cecair digunakan dalam sistem hidraulik.sistem hidraulik menggunakan cecair yang tidak dikompresikan seperti minyak atau air untuk menghantar kuasa dari satu lokasi ke lokasi yang lain dalam bendalir.                             apabila ombok ditolak kedalam sfera bulat, air dipancut keluar dengan kelajuan yang sama ke semua arah. ini menunjukkan tekanan cecair boleh dipindahkan ke seluruh cecair dengan seragam.
Catat Ulasan
Baca lagi